Canada's Homeschool

It’s safe to say that I, nor anyone I know would consider me a carpenter, or even consider me a person with carpentry skills. Sure I can hammer in a nail, and have even screwed drywall to a 2” x 4”. However, the word “Carpenter” is, and should be associated with individuals who can actually see a building project through with better than acceptable results. 

It was last summer that everything would change because I decided to bear down and build a deck. It was during the construction that math hit me straight in the face. Having taught math for years, I was almost embarrassed with how I wasn’t aware of hidden math formulas in real world applications. 

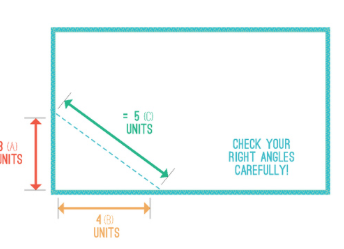
A carpenter friend had helped me with setting up the support beams, and stuck around to make sure I was going to screw in the frame correctly, meaning straight and square. While helping me balance the weight of the beams, he looks me dead in the eye and says “To make sure these are square, just use the 3, 4, 5 rule”. 

3, 4, 5 Rule 
On one side of the corner beam, he measured 3 inches from the corner and made a mark. On the opposite side of the corner, he measured 4 inches from the corner and made a mark. Next, he measured between the two marks. He then calmly looked at his measuring tape and says, “Yup, 5 inches, perfect, this corner is square, lets do the other side now.” 

I was blown away; not at that exact moment, but rather about 15 minutes later when I realized what just occurred. It wasn’t the fact that he used the numbers 3, 4 and 5, it was that my carpenter friend just used Pythagoreans Theorem to aid in the building of my deck. 

Pythagoras Theorem 
Of course! It wasn’t like I didn’t know the theorem: A2 + B2 = C2. I however always associated Pythagoreans Theorem with balancing a formula in trigonometry. I never thought of it as the 3, 4, 5 rule, and never applied it during any real life situation I had found myself in (like how far am I away from the nearest 7-11 if I have to walk 6 blocks north and then 8 blocks west? Google maps can tell me it’s 10 blocks). UGH, sine, cos, tan, you still haunt me. 

By using the Pythagorean Theorem; If we plug the following values into the theorem (a=3, b=4, c=5), we find that the equation is true: three-squared (9) plus four-squared (16) is equal to five-squared (25). 

Now to be fair, I’m sure if the shoe was on the other foot and I told him that I would simply be using Pythagoreans Theorem to make sure these corners were square, he may have looked at me in an odd fashion. He was using the theorem in his everyday work life, perhaps without even knowing what the “mathematical” term was, which is far better than knowing the “mathematical” term and 
never using it. 

Resources

http://www.mathsisfun.com/pythagoras.html
http://www.wikihow.com/Use-the-3-4-5-Rule-to-Build-Square-Cornershttps://www.youtube.com/watch?v=69cslx6ER7k